what is the sum of all numbers from 1 to 100

I am a erstwhile maths instructor and possessor of DoingMaths. I dear writing about maths, its applications and fun mathematical facts.

Carl Friedrich Gauss

Carl Friedrich Gauss (1777 - 1855)

Carl Friedrich Gauss - 'Princeps Mathematicorum'

Carl Friedrich Gauss (1777 - 1855) is one of the greatest and most influential mathematicians of all fourth dimension. He made many contributions to the fields of mathematics and science and has been referred to every bit the Princeps Mathematicorum (Latin for 'the foremost of mathematicians). However, one of the well-nigh interesting tales nearly Gauss comes from his childhood.

Calculation the Numbers From 1-100: How Gauss Solved the Problem

The story goes that Gauss'southward main school teacher, existence the lazy type, decided to go along the class occupied by getting them to sum all of the numbers from 1 - 100. With a hundred numbers to add upward (without calculators in the 18th century) the instructor idea that this would continue the grade busy for quite some time. He hadn't reckoned on the mathematical ability of immature Gauss however, who merely a few seconds later on came back with the right answer of 5050.

Gauss had realised that he could make the sum a lot easier past adding the numbers together in pairs. He added the first and the last numbers, the second and the 2d to terminal numbers and so on, noticing that these pairs i + 100, 2 + 99, three + 98, etc. all gave the same respond of 101. Going all the way to 50 + 51 gave him 50 pairs of 101 and an reply of 50 × 101 = 5050.

Summing Integers from 1 - 100 on the DoingMaths YouTube channel

Extending Gauss'southward Method to Other Sums

Whether this story is really truthful or non is unknown, but either style it gives a fantastic insight into the heed of an extraordinary mathematician and an introduction to a speedier method of adding together arithmetic sequences (sequences of numbers formed past increasing or decreasing by the same number each time).

First of all let's look at what happens for summing sequences like Gauss's, but to any given number (not necessarily 100). For this we can expand Gauss's method quite simply.

Suppose we desire to add together all of the numbers up to and including due north, where n represents whatever positive whole number. We will add together the numbers in pairs, kickoff to last, 2d to second to last then on as we did above.

Allow's use a diagram to help usa visualise this.

Summing the Numbers From 1 to n

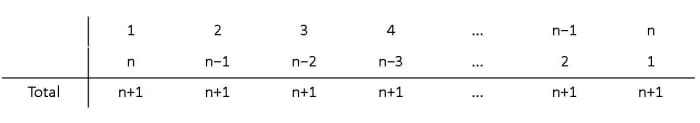
Summing the Numbers From 1 to n

By writing the number one − n and then repeating them backwards below, nosotros can see that all of our pairs add up to n + 1. There are at present n lots of north + i in our motion-picture show, but nosotros got these using the numbers one - due north twice (once frontward, i in opposite), hence to get our reply, we need to halve this total.

This gives u.s. a terminal answer of 1/2 × n(north + 1).

Using Our Formula

We can check this formula against some real cases.

In Gauss's example we had 1 - 100, so n = 100 and the total = one/two × 100 × (100 + 1) = 5050.

The numbers 1 - 200 sum to 1/ii × 200 × (200 + 1) = twenty 100 while the numbers 1 - 750 sum to 1/2 × 750 × (750 + 1) = 218 625.

Scroll to Continue

Read More From Owlcation

Expanding Our Formula

We don't have to stop in that location however. An arithmetic sequence is any sequence where the numbers increase or subtract by the same amount each time due east.thou. two, iv, 6, 8, 10, ... and eleven, 16, 21, 26, 31, ... are arithmetic sequences with increases of 2 and 5 respectively.

Suppose we wanted to sum the sequence of fifty-fifty numbers up to 60 (two, 4, 6, 8, ..., 58, 60). This is an arithemetic sequence with a difference between terms of 2.

We tin can utilize a simple diagram equally before.

Summing the Fifty-fifty Numbers up to threescore

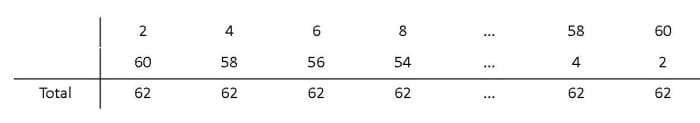
Summing the Even Numbers up to 60

Each pair adds upward to 62, but it is slightly trickier to see how many pairs we have this time. If we halved the terms 2, 4, ..., 60, we would go the sequence 1, 2, ..., thirty, hence at that place must exist thirty terms.

We therefore have 30 lots of 62 and once again, because we have listed our sequence twice, we demand to halve this so 1/2 × 30 × 62 = 930.

Creating a General Formula for Summing Arithmetic Sequences When Nosotros Know the Start and Final Terms

From our example we can meet quite chop-chop that the pairs e'er add up to the sum of the first and last numbers in the sequence. We then multiply this by how many terms there are and divide by ii to counteract the fact that nosotros have listed each term twice in our calculations.

Therefore, for any arithmetic sequence with n terms, where the starting time term is a and the last term is l we can say that the sum of the outset due north terms (denoted by Southward_n), is given by the formula:

S_northward = ane/2 × n × (a + l)

What About if the Final Term is Unknown?

We can aggrandize our formula a little further for arithmetics sequences where we know there are n terms but we don't know what the northward^thursday term (the last term in the sum) is.

E.m. discover the sum of the first xx terms of the sequence 11, 16, 21, 26, ...

For this problem, n = 20, a = xi and d (the difference between each term) = v.

We tin employ these facts to discover the terminal term l.

In that location are 20 terms in our sequence. The 2nd term is 11 plus one 5 = sixteen. The 3rd term is 11 plus ii fives = 21. Each term is 11 plus one fewer 5s than its term number i.e. the seventh term volition be 11 plus half dozen 5s and and then on. Following this design, the 20^th term must be xi plus nineteen 5s = 106.

Using our previous formula we therefore have the sum of the get-go 20 terms = 1/2 × 20 × (11 + 106) = 1170.

Generalising the Formula

Using the method higher up, we can see that for a sequence with first term a and departure d, the n ^th term is always a + (n − i) × d, i.eastward. the offset term plus ane fewer lots of d than the term number.

Taking our previous formula for the sum to north terms of South_northward = one/2 × n × (a + l), and substituting in fifty = a + (n − i) × d, we get that:

Due south_n = 1/two × n × [a + a + (n − i) × d]

which tin exist simplified to:

S_n = 1/2 × n × [2a + (n − 1) × d].

Using this formula on our previous case of summing the first twenty terms of the sequence 11, 16, 21, 26, ... gives us:

Southward_n = ane/2 × 20 × [2 × 11 + (20 − 1) × 5] = 1170 equally before.

Recap

In this commodity we have discovered iii formulae that tin can be used to sum arithmetics sequences.

For unproblematic sequences of the class ane, 2, 3, ...., n,:

S_n = 1/2 × n × (north + ane)

For whatsoever arithmetics sequence with n terms, offset term a, divergence between terms d and terminal term 50 , we can use the formulae:

S_{due north} = 1/ii × n × (a + l)

or

S_north = ane/2 × n × [2a + (n − 1) × d]

This content is accurate and true to the best of the writer'southward knowledge and is not meant to substitute for formal and individualized advice from a qualified professional.

© 2021 David

wentworthcarposept.blogspot.com

Source: https://owlcation.com/stem/How-to-Add-the-Numbers-1-100-Quickly-Summing-Arithmetic-Sequences

Share this post